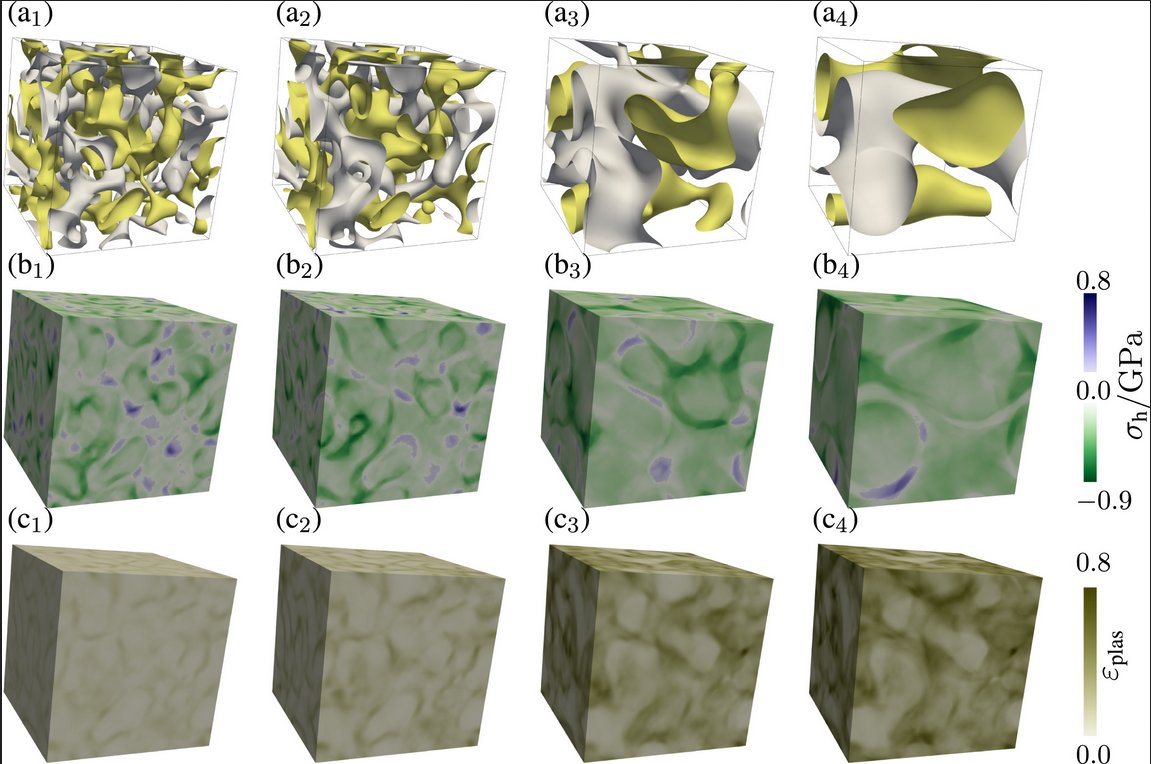
The next step in the development of phase field models for coupling mechanics, temperature and chemistry in materials modeling
The phase-field method is particularly well-suited to model coupled mechanical-thermal-chemical microstructure evolution and structure-property relations.
It has been successfully applied to model multiple thermo-chemo-mechanical processes including solidification, precipitation, fracture and dislocation motion.
Further methodological developments including chemo-mechanical interface modelling and homogenisation have also been treated.
However, the majority of chemo-mechanical phase-field modeling studies are limited to linear elastic mechanics.
Until very recently, only few works have been carried out to fully couple the chemo-elasto-plastic processes in a thermodynamically consistent framework.
The use of diffuse-interface models to describe interfacial phenomena dates back to Cahn and Hilliard, and further to Ginzburg and Landau.
The original Cahn–Hilliard equation was used to model spinodal decomposition in binary alloys, but has since been extended to multi-component systems and coupled with microelasticity.
A critical challenge in simulating the thermodynamics of multi-component chemo-mechanical systems is the numerical approximation of a generally non-convex chemical free energy.
Several numerical methods have been developed to solve non-convex systems using finite difference, mixed finite element with composition and chemical potential treated as independent fields, isogeometric and spectral spatial discretisations.
In the context of numerical time-integration, the stability, robustness and efficiency of the resulting solution algorithm is sensitive to the degree of non-convexity of the chemical free energy.
A practical and quite successful approach in this regard is the splitting of the chemical free energy into concave and convex components, which are then approximated separately.
In particular, splitting methods have recently been applied to the multi-component, multi-phase Cahn–Hilliard equation with a generalised non-convex Landau energy landsacpe.
In the context of a mixed finite-element-based weak formulation of the classic Cahn–Hilliard relation, Gomez and Hughes employed a chemical energy splitting approach based on the sign of the fourth-order derivatives of the underlying energy functional. A recent mixed weak formulation of chemo-mechanics for two-phase, two-component finite-deformation gradient elastic solids based on unconditionally stable, second-order accurate time-integration and Taylor expansion of the non-convex energy has been given by Sagiyama et al..
More recently, starting from a grand potential functional, the transport relations have been formulated for multi-component and multi-phase systems.
In such an approach, the thermodynamics of the system is set in a grand canonical ensemble and formulated in terms of the chemical potential. As a result, boundary and interface conditions based on the chemical potential, needed for a realistic representation of macroscopic systems where the total number of particles cannot be fixed, are naturally incorporated. However, these approaches are based on a Legendre transformation, which does not exist for more general non-convex forms of the chemical free energy.
In our approach, a convex splitting of the chemical free energy is instead used to invert the multi-component chemical potential–composition relation.
This results in an expression for component transport in terms of the chemical potential rather than the chemical composition, analogous to the grand potential approach, but applicable to more general forms of the chemical free energy.